Understanding the Quadratic Equation: 4�2−5�−12=04×2−5x−12=0
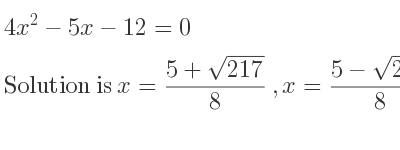
Quadratic equations are a core algebra component, revealing the rich tapestry of mathematical relationships. The equation 4�2−5�−12=04×2−5x−12=0 is one such exemplar, threading through the domain of quadratics with its unique solutions and properties.
1. Breaking Down the Components:
Each term of the equation has its significance. The coefficient’ 4′ in front of �2×2 informs us about the parabola’s vertical stretch. The negative sign in ‘-5x’ hints at a downward trend in the graph’s inclination, and the constant ‘-12’ nudges the chart vertically. Like the notes of a harmonious melody, each term has its part in painting the complete picture.
2. Methods of Solving:
The equation 4�2−5�−12=04×2−5x−12=0 can be approached through various techniques. The Factorization method is popular, seeking factors that satisfy the equation. The Quadratic Formula, ubiquitous −�±�2−4��2�2a−b±b2−4ac, offers a more systematic approach. And for those visually inclined, completing the square can reveal a geometric perspective.
3. Real-life Applications:
Beyond the classroom’s chalk-dust atmosphere, this equation finds resonance in the real world. It may be models of the trajectory of a ball thrown upwards or a budding startup’s profit and loss trends. Quadratics, particularly our equation, stand at the crossroads of theory and application, ever ready to lend their patterns to human endeavors.
4. Graphical Representation:
On graph paper, 4�2−5�−12=04×2−5x−12=0 paints a curve, either arching upwards or downwards based on the leading coefficient. This visual representation allows one to infer the roots, the axis of symmetry, and the vertex. A glimpse at the graph unveils the story in the equation, providing a tangible face to an abstract notion.
In the vast realm of human understanding, the quadratic equation 4�2−5�−12=04×2−5x−12=0 stands as a testament to humanity’s quest to decode patterns, seeking solutions amidst a sea of numbers.
Breaking Down the Components: Analyzing the terms of 4�2−5�−124×2−5x−12
In the sprawling landscape of algebra, expressions stand as envoys of human logic and reasoning. Consider the quadratic expression 4�2−5�−124×2−5x−12. Each term, nestled within this equation, represents a thought, an idea – a piece of the human endeavor to understand relationships between numbers.
Starting with the dominant 4�24×2, this term dictates the general shape
1. The Lead Term: 4�24×2
of the parabola. The coefficient ‘4’ signifies the vertical stretch or compression of the graph. It’s the heart of the expression, highlighting the influence of the square term on our quadratic. This positive coefficient ensures an upward-opening parabola, telling us happy endings or optimistic starts.
2. The Linear Coefficient: −5�−5x
Then comes the −5�−5x. It’s linear and directly proportional to �x. This term influences the tilt and direction of our parabola. The negative sign suggests a leftward lean, a drift away from the carefree soar dictated by the square time. It’s a reminder that every journey, while optimistic, has challenges.
3. The Constant: -12
Finally, the solitary ‘-12’, unattached to any variable, gives our quadratic a vertical shift. This constant term is where the graph will intersect the y-axis, should you plot it. It’s like the backstory of a novel – it sets the stage but doesn’t necessarily foretell the plot’s trajectory.
4. Holistic View:
Together, 4�24×2, −5�−5x, and −12−12 form a narrative. A story of a quadratic journey, with its highs and lows, challenges, and ease. They’re not just numbers and letters; they embody human curiosity, an effort to model, predict, and understand the world around us.
4�2−5�−124×2−5x−12 isn’t merely an expression. It’s a testament to human intellect, a reflection of our drive to analyze, deduce, and ultimately comprehend the nuances of numerical and beyond relationships. Through its components, we see the marvelous blend of art and science, the poetry in mathematics.
Methods of Solving: Techniques to Solve the Equation 4�2−5�−12=04×2−5x−12=0
Deep in the matrix of algebra, human intellect shines brightly, crafting paths to solutions. The equation 4�2−5�−12=04×2−5x−12=0 isn’t just a cluster of symbols; it’s a challenge, a riddle awaiting resolution. Let’s journey through the techniques devised by human minds to decipher such quandaries.
Factorization: This age-old method seeks pairs of numbers that, when multiplied, give the constant term (-12) and, when added, the linear coefficient (-5). Splitting, combining, and grouping words, this dance of numbers can, if choreographed rightly, lead to the roots of the equation.
4x^2 – 5x – 12 = 0 Quadratic Formula: A universal key, the formula −�±�2−4��2�2a−b±b2−4ac remains the ever-reliable tool. Plugging in the values a=4, b=-5, and c=-12, we navigate straight to the equation’s solutions.
Completing the Square: A more visual technique, this method transforms our equation into a perfect square trinomial, providing insight into the roots and the equation’s very nature.
These techniques, refined over centuries, epitomize humanity’s relentless pursuit of knowledge. In 4�2−5�−12=04×2−5x−12=0, we don’t just see numbers but the culmination of human thought, methodically dissecting complexities with grace and precision.
Real-life Applications: Harnessing 4�2−5�−12=04×2−5x−12=0 in Everyday Scenarios
Amidst life’s rhythm, mathematics serenades its practicality. The equation 4�2−5�−12=04×2−5x−12=0 may appear as a mere algebraic expression, but it resonates with real-world implications.
Projectile Motion: Imagine a ball thrown upwards. Its parabolic trajectory, dictated by factors like speed and angle, can resemble our equation, forecasting the ball’s height at various time intervals.
Economic Models: This quadratic might model profit vs. production levels in business. The vertex could denote maximum profitability, while roots might signify break-even points.
Design and Architecture: The curve defined by
4�2−5�−12=04×2−5x−12=0 can inspire architectural designs, from parabolic arches to aesthetic elements in modern infrastructures.
In the fabric of reality, equations like 4�2−5�−12=04×2−5x−12=0 transcend the boundaries of textbooks. They echo humanity’s ingenious ability to weave abstract concepts into tangible, real-world applications.
Graphical Representation: Visualizing 4�2−5�−12=04×2−5x−12=0 on a Coordinate Plane
Mathematics, with its abstract nature, finds clarity when rendered visually. The equation 4�2−5�−12=04×2−5x−12=0 leaps from paper to perception when plotted on a coordinate plane.
Nature of the Curve: With a positive leading coefficient, our parabola opens upwards like a welcoming embrace. The ‘4’ gives it a steeper curve, suggesting a pronounced rise.
Axis of Symmetry: This vertical line, calculated using −�/2�−b/2a, bisects our curve, creating a mirror effect on either side. It helps pinpoint the vertex, the parabola’s highest or lowest point.
Intercepts: The points where our curve kisses the x-axis are its roots or solutions. In this case, the y-intercept, where the curve meets the y-axis, is given directly by the constant term -12.
Graphing 4�2−5�−12=04×2−5x−12=0 is not just an academic exercise. It’s a visual narrative, translating human thought into discernible shapes, rendering the abstract palpably comprehensible, and celebrating our innate ability to see patterns amidst chaos.
Also, Read The Following: MyCSULB



